

Issac Newton's mathematics of "fluxions" was the first form of differential calculus. that the first exact theory of infinitesimals was developed 300 years after the invention of differential calculus.Ībraham Robinson (1918-1974), Incompleteness, The Proof and Paradox of Kurt Gödel, by Rebecca Goldstein In coming centuries it will be considered a great oddity. a fad of mathematical logicians the analysis of the future.

John Stillwell, Yearning for the Impossible, The Surprising Truths of Mathematics, pp.99-100 However, nonstandard analysis is not yet as simple as the old Leibniz calculus of infinitesimals, and there is a continuing search for a really natural system that uses infinitesimals in a consistent way.
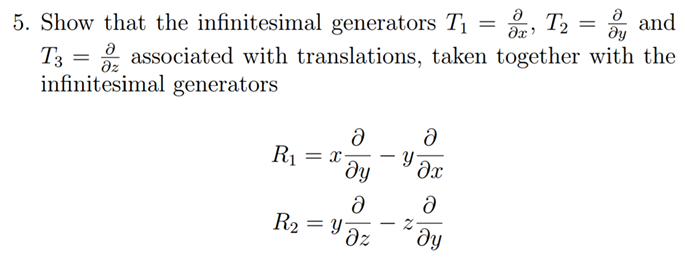
His system is called nonstandard analysis, and it has been successful enough to yield some new results. The first to solve this problem completely was the American mathematician Abraham Robinson, in the 1960s. Is it possible to define and use genuine infinitesimals?. To many this is a compromise solution which fails to explain why infinitesimals work. Thus avoidance of infinitesimals came at the cost of a strange dual notation: Δ for actual differences and d (the ghost of Leibniz!) for the limits of their quotients and sums. Likewise, ∫ y dx is not an actual sum of terms y dx, but the limit of a sum of terms y Δ x. It has to be explained that dy/dx is not the ratio of infinitesimal differences dy and dx - since infinitesimals do not exist - but is rather a symbol for the limit of the ratio Δ y/Δ x as Δ x tends to zero, where Δ x is a finite change in x and Δ y is the corresponding change in the function y of x. For example "let dx be infinitesimal" would be restated as "let Δ x tend to zero." However, even the mainstream approach uses the Leibniz notations dy/dx and ∫ y dx, because they are so concise and suggestive. It denies the existence of infinitesimals, and intreprets the word "infinitesimal" as a mere figure of speech in statements that are properly made using limits. This is the mainstream approach to calculus used today. between 18 a serviceable approach to calculus was worked out, based on the concepts of function and limit. Yet we cannot show him a point without extension or a line without breadth hence we can just as little explain to him the a priori nature of mathematics as the a priori nature of right, because he pays no heed to any knowledge that is not empirical.Īrthur Schopenhauer, The World as Will and Representation, Volume I, §62, p.342 Hobbes characterizes his completely empirical way of thinking very remarkably by the fact that, in his book De Principiis Geometrarum, he denies the whole of really pure mathematics, and obstinately asserts that the point has extension and the line breadth. wie wir dem selben Hobbes, der jene seine vollendet empirische Denkungsart höchst merkwürdig dadurch charakterisirt, daß er in seinem Buche ✽e principiis Geometarum« die ganze eigentlich reine Mathematik ableugnet und hartnäckig behauptet, der Punckt habe Ausdehnung und die Linie Breite, doch nie einen Punkt ohne Ausdehnung und eine Linie ohne Breite vorzeigen, also ihm so wenig die Apriorität der Mathematik, als die Apriorität des Rechts beibringen können, weil er sich nun ein Mal jeder nicht empirischen Erkenntniß verschließt. Philosophical Problems with Calculus Philosophical Problems


 0 kommentar(er)
0 kommentar(er)
